Introduction to VISUALIZING Einstein's Relativity

WHY VISUALIZATION IS IMPORTANT

Although the he could "do the math", Albert Einstein relied on VISUAL ANALOGIES for his greatest insights that revolutionized the world of science.

Let me share some of his  insights (and a couple of mine) that may help you better VISUALIZE and even understand a bit of Einstein's Theories of Special and General Relativity.  (Click here to download the PowerPoint charts I used to presented some of this material to the Philosophy Club of The Villages, FL, on 31 July 2015.)

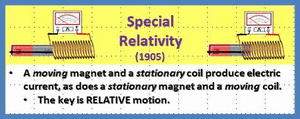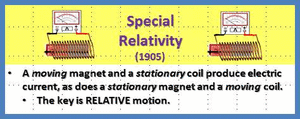
For example, in his famous 1905 paper that introduced what has come to be called the theory of  "Special Relativity", Einstein starts with a simple VISUALIZATION, depicted in my graphic below.

Prior to attending Zurich Polytechnic, where he earned a degree in Mathematics and Physics, Einstein had worked with his father who owned an electrical equipment business. Therefore, young Albert had some practical ENGINEERING experience as a foundation upon which he could "do the MATH" and create the PHYSICS theories that revolutionized our understanding of the workings of the Universe, and made him famous.

He knew, from practical experience working with his father on electrical equipment, that a stationary coil of wire would generate an electric current if a magnet was moved back and forth within it. He also knew the reverse was true. A stationary magnet would generate an electric current in a coil of wire if the coil was moved back and forth over the magnet. The key, of course, was the RELATIVE movement of the coil and the magnet.

VISUALIZATION AS AN AID TO CREATIVITY AND UNDERSTANDING

I agree with Einstein that there are (relatively :^) simple descriptions that can help "even a child" understand "all physical theories" (apart from their mathematical expressions).

The key to this level of understanding is VISUALIZATION aids, such as the graphic above and the ones I will use in this series of Blog posts.

Yes, of course the mathematics is important for a full understanding, but, as an ENGINEER (and not a PHYSICIST), I tend to approach science and technology via the VISUALIZATION route first.

My bachelors degree is in Electrical Engineering (1961) and my Masters (1990) and PhD (1996) are in System Science, so I have some academic ability to understand physics and "do the math". During my long, creative, and successful engineering career (at IBM and Lockheed-Martin) I collaborated with some brilliant mathematicians and physicists, along with system, hardware and software engineers without whom my creative concepts would never have seen the light of day.

It seems to me that (at least some) physicists, including some who are (justifiably) well recognized and honored for their contributions to science, get confused and even mislead by the mathematics.

For example, it is common for physicists to say that some physical reaction is "governed by this equation". NOPE! The physical reaction is in accordance with the Laws of Nature, and our equations (and, now, computer models) are mere approximations of actual reality. As my PhD adviser (Howard Pattee, a physicist) taught me, "the map is not the territory!"

OK, back to Einstein's Relativity!

ESSENCE OF EINSTEIN'S THEORY OF SPECIAL RELATIVITY (SR)

The graphic depicts a moving observer A who is on a train going at a substantial fraction of the speed of light, and a stationary observer B who is at a station that is the same length as the train. Both observers have identical clocks, flashing beacons, and instruments they may use to measure clock rates, the length of the train and the station, and the speed of the light emanating from the flashing beacons.

Starting with the idea that relative motion is the key to generation of electricity, Einstein takes a gigantic leap of insightful imagination and states, as a postulate, that the speed of light (in a vacuum) is constant for all observers, no matter the speed of the source or of the observer. Einstein referred to his concept of the measured speed of light being constant as "invariability".

Thus, observers A and B will measure the exact same speed of light for the light emanating from their own as well as the other's flashing beacons, no matter how fast they, or the other, is moving.

NOTE THAT THIS IS COUNTER-INTUITIVE. For example, if observer A, on the train, fired a gun towards the station, the speed of the bullet, as measured by stationary observer B, would be the muzzle velocity PLUS the train velocity. If observer B fired a gun and measured the speed of the bullet, it would be just the muzzle velocity. So, for bullets fired from guns, observer B would measure a greater speed for bullets from observer A's gun than from her own. Not so with a light beam, according to Einstein (and he turned out to be correct).

Furthermore, observer B will measure the length of the train as being contracted (that is, shorter than the length of her station) and the rate of the clock on the train being dilated (that is, slower than the clock at her station).

Perhaps surprisingly, observer A will measure the length of the station as being contracted (that is, shorter than the length of his train) and the rate of the clock at the station being dilated (that is, slower than the clock on his train).

So, each measures the length and clock rates of the other as being less than their own! How can that be? Well, an internet site suggests it is like two cars passing on a road. When the drivers look into their rear view mirrors, the other car appears to be getting smaller. Of course, we know that both cars are the same length, regardless of what the drivers observe in their rear-view mirrors.

However, according to Einstein's Special Relativity (and all experiments to date), one of the two bodies in relative motion (in this case the train or the station) actually does have its length contracted and clock speed reduced relative to the other. Which one? Stay tuned and you will find out. (HINT: If you think it is the train that actually experiences relativistic effects in this case, you are correct!)

ESSENCE OF EINSTEIN'S THEORY OF GENERAL RELATIVITY (GR)

Special Relativity applies only to bodies in relative motion at constant speed. In 1915, Einstein extended his theory to the more general case of accelerating bodies, that is bodies that change speed or turn during observation.

The graphic depicts two scientists, equipped with identical instruments. who are confined to sealed boxes.

One scientist is in a sealed  box on Earth, where GRAVITY is 32.2 feet per second squared (9.8 meters per second squared).

The other is in Deep Space, far from any massive bodies, on a rocket that is ACCELERATING at the rate of 32.2 feet per second squared.

Einstein reasoned that the scientists could not tell whether they were on Earth (experiencing GRAVITY) or in Deep Space (experiencing ACCELERATION).

From this basic insight, Einstein concluded that the relativistic effects of GRAVITY were equivalent to the relativistic effects of ACCELERATION. He also "pictured" the effect of a massive body on SpaceTime as causing it to "curve".

HOW ARE SPECIAL RELATIVITY AND GENERAL RELATIVITY  RELATED?

^{_{In my research for this project, I happened upon a fact that is not prominently mentioned by many Internet expositions of Relativity. Namely that:}}

 Time Dilation, TD, due to General Relativity
at a location near a massive body 

is exactly equal to 

the TD due to Special Relativity for a spacecraft 

(in deep space far from any massive body) 

moving at the Escape Velocity 

corresponding to that location!

At any location near a massive sphere, Escape Velocity is the speed at which the Kinetic Energy is exactly equal and opposite to Gravitational Potential Energy, such that a rocket moving at that speed will continue out to space forever without any need for further propulsion. 

Kinetic ENERGY, KE is LINEARLY related to TD. An increase (or decrease) in KE will result in an exactly proportional increase (or decrease) in TD.

Speed is NOT linearly related to TD. At low speeds, a given increase in speed results in a minuscule increase in TD. However, at high speeds (a substantial fraction of the speed of light), the same increase in speed results in a large increase in TD. 

Gravitational Potential ENERGY, GPE is LINEARLY related to TD. An increase (or decrease) in GPE will result in an exactly proportional increase (or decrease) in TD.

Gravity, g, per se, is NOT linearly related to TD.  Indeed, it is NOT even monotonic. At the center of a massive object, where TD is maximized, g is zero. At the surface, where g reaches its maximum (negative) value, TD is less than at the center, but more than at infinity. At an infinity, where TD is zero, g is also zero. The fact that the relationship is non-monotonic is clear because within a massive object, smaller levels of g are associated with increasing TD. Outside a massive object, smaller levels of g are associated with decreasing TD. 

When you throw a ball straight up into the air at some initial vertical speed, it will not continue to go up forever. As it rises it will slow its upward speed until it reaches the point where its speed is zero. Then it falls, continuously increasing downward speed, until it returns to your glove. If we ignore air friction, the ball will strike your glove at exactly the same speed as your initial throw.

This is a perfect illustration of the exchange of Kinetic Energy for Gravitational Potential Energy. Your initial throw imparts a given vertical speed to the ball. From that speed, you can compute the KE. As the ball rises and slows due to the force of Gravity, the KE is converted to GPE (ignoring loss to air friction). At the highest point, the ball has zero Kinetic Energy, and maximum GPE relative to your glove. By conservation of Energy, the GPE at the peak is exactly equal to the initial KE of the throw. As the ball falls, the process is reversed, with the GPE being converted to KE. 

(NOTE: By convention, GPE is considered negative - because it points downward towards the massive object that is the source of the gravitational field. GPE is zero at an infinite distance, and gains in magnitude (becomes more negative) closer to the massive object. KE is considered positive. So, in the preceding paragraph, when I say that the GPE at the highest point is exactly equal to the initial KE of the throw, what actually happens is that, as the ball rises and slows, KE goes from some high positive value to zero (reduces), while GPE goes from some high negative value to a less negative value (increases). The reduction in KE is exactly equal to the increase in GPE. Keep that in mind as you read the following.) 

Is there a speed at which you could throw that ball such that it will keep going up forever? In other words, could you give it the KE that corresponds to the GPE of a ball that is infinitely far from Earth? The answer is YES, and that speed is called the Escape Velocity from Earth. Of course, you could not throw a ball that fast, but you could if you had a rocket!

Escape Velocity from the Earth Surface is about 25,000 MPH (40,000 km/hr). It is defined as the launch speed required for a spacecraft, pointing straight up, such that it will have just enough KE so it will not fall back to Earth (ignoring air friction and rotation of the Earth).

The graphic illustrates the equivalence between the effects of GRAVITY (GENERAL RELATIVITY - on the left) and SPEED (SPECIAL RELATIVITY - on the right). 

An observer "at rest" on the Earth will experience relativistic effects (length contraction and time dilation) due to his GPE with respect to infinity. That GPE is the energy required to move an object from the surface of the Earth to infinity. 

At that level of GPE, it would take an equivalent level of KE for a rocket to leave the Earth and never return. That level of KE corresponds to the Escape Velocity from the Earth's surface.

An observer in a rocket in Deep Space, far from any massive object and moving at 25,000 MPH will experience exactly the same relativistic effects (length contraction and time dilation) due to her KE with respect to the Earth as the "at rest" rocket on Earth. 

PUTTING IT ALL TOGETHER - JUMP INTO A TUNNEL THROUGH THE EARTH

In the above graphic, POTENTIAL ENERGY is with respect to the center of the Earth, and is related to, but NOT the same, as Gravitational Potential Energy, GPE.
For good (but confusing) reasons, GPE is always ZERO or NEGATIVE.
  GPE(Infinity) is defined as ZERO. GPE(Surface) and GPE(Center) are defined as the work (energy) required to raise an object from the Center or the Surface to Infinity. The magnitude of GPE(Center) is larger than the magnitude of GPE(Surface), however, since both are negative quantities, GPE(Center), on the real number scale, is numerically LESS than GPE(Surface).
The POTENTIAL ENERGY in the above graphic is the GPE at a given location in the tunnel (either GPE(Surface) or GPE(Midway) MINUS GPE(Center), and is therefore a positive quantity. 

Consider three observers:

A is "at rest" on the surface of the Earth. He has zero SPEED, so he has zero KE. But, what is his POTENTIAL ENERGY, PE(Surface)? Since POTENTIAL ENERGY is with respect to the Center, it is GPE(Surface), MINUS GPE(Center), a POSITIVE quantity. At the Surface, Gravity, g = 32.2 feet per second squared.

B is "at rest" in the very center of the Earth. He has zero SPEED, so he has zero KE. But, what is POTENTIAL ENERGY. PE(Center)? Since POTENTIAL ENERGY is with respect to the Center, it is GPE(Center), MINUS GPE(Center), which is ZERO. At the Center, g = 0.0 feet per second squared.

C starts "at rest" on the surface of the Earth. At that point, She has zero SPEED, so she has zero KE. And, like A, she PE(surface), a POSITIVE Quantity. At the Surface, Gravity, g = 32.2 feet per second squared.

C jumps into the tunnel!  She falls at increasing SPEED, so her KE increases. By conservation of energy, assuming a friction-free tunnel, her PE must decrease by the same amount as her KE increases. So, when she is about halfway from the surface to the center, she has medium KE and medium PE. Since GRAVITY decreases linearly within a spherical mass, at the midway point, g = 16.1 feet per second squared.

C comes to the center of the Earth! At this point, she is moving very fast, so she has maximum KE. However, being at the center of the Earth, she zero PE with respect to the Center. At the Center, g = 0.0 feet per second squared.

C continues towards the other side of the Earth, g increases in magnitude from zero towards its eventual value of -32.2 feet per second squared at the surface, her SPEED decreases, and her KE decreases. So, at this point, she has medium KE and PE. 

C reaches the other side of the Earth. At this point (but only momentarily) she is again "at rest"  on the surface of the Earth. At that point, She has zero SPEED, so she has KE = 0, and PE(Surface). 

So what happens? C falls back into the hole, and repeats the process of exchanging PE and KE. If the hole is friction-free, she neither gains nor looses net Energy (the simple sum of PE PLUS KE). This process will continue forever, and it turns out that the journey from top Surface to bottom Surface is about 42 minutes, or about 84 minutes for the round trip.

Please notice that C will experience the relativistic effects of Special Relativity plus the relativistic effects of General Relativity when passing through the center of the Earth. When momentarily "at rest" at the surface, she will experience only the relativistic Effects of General Relativity. Thus, her TD will be greater at the center due to more KE and also greater at the center due to more GPE. 

CONCLUSION

So, please click on each of the RELATIVITY TOPICS listed at the top of the right-hand column under the Blog title.

advTHANKSance !

Ira Glickstein